Exam 3: The Derivative and the Tangent Line Problem
Exam 1: Graphs and Models114 Questions
Exam 2: A Preview of Calculus92 Questions
Exam 3: The Derivative and the Tangent Line Problem191 Questions
Exam 4: Extrema on an Interval147 Questions
Exam 5: Antiderivatives and Indefinite Integration167 Questions
Exam 6: Slope Fields and Eulers Method85 Questions
Exam 7: Area of a Region Between Two Curves120 Questions
Exam 8: Basic Integration Rules127 Questions
Exam 9: Sequences179 Questions
Exam 10: Conics and Calculus120 Questions
Exam 11: Vectors in the Plane125 Questions
Exam 12: Vector-Valued Functions83 Questions
Exam 13: Introduction to Functions of Several Variables124 Questions
Exam 14: Iterated Integrals and Area in the Plane118 Questions
Exam 15: Vector Fields108 Questions
Exam 16: Exact First-Order Equations45 Questions
Select questions type
A conical tank (with vertex down) is feet across the top and feet deep. If water is flowing into the tank at a rate of cubic feet per minute, find the rate of change of the Depth of the water when the water is feet deep.
(Multiple Choice)
4.8/5  (40)
(40)
Suppose the position function for a free-falling object on a certain planet is given by . A silver coin is dropped from the top of a building that is 1372 feet tall. Find the instantaneous velocity of the coin when .
(Multiple Choice)
4.8/5  (31)
(31)
airplane flies at an altitude of 14 miles toward a point directly over an observer. Consider and as shown in the following figure. Write as a function of .
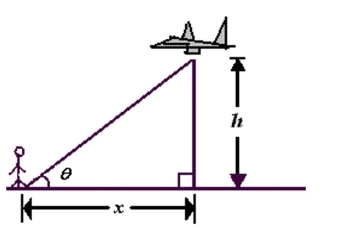
(Multiple Choice)
4.8/5  (33)
(33)
The displacement from equilibrium of an object in harmonic motion on the end of a spring is where is measured in feet and is the time in seconds. Determine the position of the object when . Round your answer to two decimal places.
(Multiple Choice)
4.7/5  (26)
(26)
Approximate the positive zero(s) of the function places. Use Newton's Method and continue the process until two successive approximations differ by less than 0.001.
(Multiple Choice)
4.8/5  (28)
(28)
Suppose the position function for a free-falling object on a certain planet is given by . A silver coin is dropped from the top of a building that is 1370 feet tall. Determine the velocity function for the coin.
(Multiple Choice)
4.8/5  (33)
(33)
Suppose the position function for a free-falling object on a certain planet is given by . A silver coin is dropped from the top of a building that is 1372 feet tall. Find the time required for the coin to reach ground level. Round your answer to the three decimal places.
(Multiple Choice)
4.8/5  (40)
(40)
decimal places. (A fixed po int of a function is a value of such that .)
(Multiple Choice)
4.7/5  (21)
(21)
A ball is thrown straight down from the top of a 300-ft building with an initial velocity of per second. The position function is . What is the velocity of the ball after 4 seconds?
(Multiple Choice)
4.9/5  (40)
(40)
Showing 21 - 40 of 191
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)