Question 1

$\text { Find } \frac { d y } { d x } \text { by implicit differentiation. }$ $x ^ { \frac { 6 } { 7 } } + y ^ { \frac { 8 } { 5 } } = 9$

Accepted Answer

A) $\frac { d y } { d x } = - \frac { 28 x ^ { \frac { - 1 } { 7 } } } { 15 y ^ { \frac { 3 } { 5 } } }$ 
B) $\frac { d y } { d x } = - \frac { 15 x ^ { \frac { - 1 } { 7 } } } { 7 y ^ { \frac { 3 } { 5 } } }$ 
C) $\frac { d y } { d x } = - \frac { 3 x ^ { \frac { - 1 } { 7 } } } { 28 y ^ { \frac { 3 } { 5 } } }$ 
D)  $\frac { d y } { d x } = \frac { 15 x ^ { \frac { - 1 } { 7 } } } { 28 y ^ { \frac { 3 } { 5 } } }$ 
E) $\frac { d y } { d x } = - \frac { 15 x ^ { \frac { - 1 } { 7 } } } { 28 y ^ { \frac { 3 } { 5 } } }$ 
A) $\frac { d y } { d x } = - \frac { 28 x ^ { \frac { - 1 } { 7 } } } { 15 y ^ { \frac { 3 } { 5 } } }$ 
B) $\frac { d y } { d x } = - \frac { 15 x ^ { \frac { - 1 } { 7 } } } { 7 y ^ { \frac { 3 } { 5 } } }$ 
C) $\frac { d y } { d x } = - \frac { 3 x ^ { \frac { - 1 } { 7 } } } { 28 y ^ { \frac { 3 } { 5 } } }$ 
D)  $\frac { d y } { d x } = \frac { 15 x ^ { \frac { - 1 } { 7 } } } { 28 y ^ { \frac { 3 } { 5 } } }$ 
E) $\frac { d y } { d x } = - \frac { 15 x ^ { \frac { - 1 } { 7 } } } { 28 y ^ { \frac { 3 } { 5 } } }$

Question 2

Find the derivative of the function. $$f ( t ) = ( 1 + 8 t ) ^ { \frac { 5 } { 9 } }$$

Accepted Answer

A) $f ^ { t } ( t ) = \frac { 40 } { 9 } ( 1 + 8 t ) ^ { \frac { - 4 } { 9 } }$ 
B) $f ^ {t } ( t ) = \frac { 8 } { 9 } ( 1 + 8 t ) ^ { \frac { - 4 } { 9 } }$ 
C) $f ^ { t } ( t ) = \frac { 1 } { 9 } ( 1 + 8 t ) ^ { \frac { - 4 } { 9 } }$ 
D) $f ^ { t } ( t ) = \frac { 40 } { 9 } ( 1 + 8 t ) ^ { \frac { - 4 } { 5 } }$ 
E) $f ^ { t } ( t ) = 8 ( 1 + 8 t ) ^ { \frac { - 4 } { 9 } }$ 
A) $f ^ { t } ( t ) = \frac { 40 } { 9 } ( 1 + 8 t ) ^ { \frac { - 4 } { 9 } }$ 
B) $f ^ {t } ( t ) = \frac { 8 } { 9 } ( 1 + 8 t ) ^ { \frac { - 4 } { 9 } }$ 
C) $f ^ { t } ( t ) = \frac { 1 } { 9 } ( 1 + 8 t ) ^ { \frac { - 4 } { 9 } }$ 
D) $f ^ { t } ( t ) = \frac { 40 } { 9 } ( 1 + 8 t ) ^ { \frac { - 4 } { 5 } }$ 
E) $f ^ { t } ( t ) = 8 ( 1 + 8 t ) ^ { \frac { - 4 } { 9 } }$

Question 3

Find the points at which the graph of the equation has a vertical or horizontal tangent line. $$5 x ^ { 2 } + 4 y ^ { 2 } - 10 x + 24 y + 8 = 0$$

Accepted Answer

A)  There is a vertical tangent at $y = - 3$ but no horizontal tangents. 
B)  There is a horizontal tangent at $x = 1$ and a vertical tangent at $y = - 3$. 
C)  There is a horizontal tangent at $x = 1$ but no vertical tangents. 
D)  There is a horizontal tangent at $x = - 2$ and a vertical tangent at $y = - 2$ 
E)  There are no horizontal or vertical tangent lines. 
A)  There is a vertical tangent at $y = - 3$ but no horizontal tangents. 
B)  There is a horizontal tangent at $x = 1$ and a vertical tangent at $y = - 3$. 
C)  There is a horizontal tangent at $x = 1$ but no vertical tangents. 
D)  There is a horizontal tangent at $x = - 2$ and a vertical tangent at $y = - 2$ 
E)  There are no horizontal or vertical tangent lines.

Question 4

The radius r of a sphere is increasing at a rate of inches per minute. Find the rate of change of the volume when $r = 11 \text { inches. }$

Accepted Answer

A)  $\frac { d V } { d t } = 3630 \pi \mathrm { in } ^ { 3 } / \mathrm { min }$ 
B)  $\frac { d V } { d t } = 1452 \pi \mathrm { in } ^ { 3 } / \mathrm { min }$ 
C)  $\frac { d V } { d t } = 2904 \pi \mathrm { in } ^ { 3 } / \mathrm { min }$ 
D)  $\frac { d V } { d t } = \frac { 1 } { 1452 \pi } \mathrm { in } ^ { 3 } / \mathrm { min }$ 
E)  $\frac { d V } { d t } = \frac { 1 } { 2904 \pi } \mathrm { in } ^ { 3 } / \mathrm { min }$ 
A)  $\frac { d V } { d t } = 3630 \pi \mathrm { in } ^ { 3 } / \mathrm { min }$ 
B)  $\frac { d V } { d t } = 1452 \pi \mathrm { in } ^ { 3 } / \mathrm { min }$ 
C)  $\frac { d V } { d t } = 2904 \pi \mathrm { in } ^ { 3 } / \mathrm { min }$ 
D)  $\frac { d V } { d t } = \frac { 1 } { 1452 \pi } \mathrm { in } ^ { 3 } / \mathrm { min }$ 
E)  $\frac { d V } { d t } = \frac { 1 } { 2904 \pi } \mathrm { in } ^ { 3 } / \mathrm { min }$

Question 5

$\text { Find the derivative of the function } y = \ln \left( x \sqrt { x ^ { 2 } + 7 } \right) \text {. }$

Accepted Answer

A)  $\frac { x } { \sqrt { x ^ { 2 } + 7 } } \ln ( x ) + \frac { 1 } { x } \ln \left( \sqrt { x ^ { 2 } + 7 } \right)$ 
B)  $\frac { 1 } { x \sqrt { x ^ { 2 } + 7 } }$ 
C)  $\frac { 1 } { x } + \frac { 1 } { \sqrt { x ^ { 2 } + 7 } }$ 
D)  $\frac { 1 } { x } + \frac { x } { x ^ { 2 } + 7 }$ 
E)  $\frac { x } { x + \sqrt { x ^ { 2 } + 7 } }$ 
A)  $\frac { x } { \sqrt { x ^ { 2 } + 7 } } \ln ( x ) + \frac { 1 } { x } \ln \left( \sqrt { x ^ { 2 } + 7 } \right)$ 
B)  $\frac { 1 } { x \sqrt { x ^ { 2 } + 7 } }$ 
C)  $\frac { 1 } { x } + \frac { 1 } { \sqrt { x ^ { 2 } + 7 } }$ 
D)  $\frac { 1 } { x } + \frac { x } { x ^ { 2 } + 7 }$ 
E)  $\frac { x } { x + \sqrt { x ^ { 2 } + 7 } }$

Question 6

$\text { Evaluate the derivative of the function } f ( t ) = \frac { 8 t ^ { 2 } + 2 } { 5 t - 1 } \text { at the point } \left( 4 , \frac { 130 } { 19 } \right) \text {. }$

Accepted Answer

A)  $f ^ { t } ( t ) = \frac { 566 } { 6859 }$ 
B)  $f ^ { t } ( t ) = - \frac { 566 } { 361 }$ 
C)  $f ^ { \t } ( t ) = \frac { 566 } { 19 }$ 
D)  $f ^ { t } ( t ) = - \frac { 566 } { 19 }$ 
E)  $f ^ { t } ( t ) = \frac { 566 } { 361 }$ 
A)  $f ^ { t } ( t ) = \frac { 566 } { 6859 }$ 
B)  $f ^ { t } ( t ) = - \frac { 566 } { 361 }$ 
C)  $f ^ { \t } ( t ) = \frac { 566 } { 19 }$ 
D)  $f ^ { t } ( t ) = - \frac { 566 } { 19 }$ 
E)  $f ^ { t } ( t ) = \frac { 566 } { 361 }$

Question 7

Use implicit differentiation to find an equation of the tangent line to the ellipse $$\frac { x ^ { 2 } } { 2 } + \frac { y ^ { 2 } } { 98 } = 1 \text { at } ( 1,7 )$$

Accepted Answer

A)  $y = - 11 x + 11$ 
B)  $y = - 2 x + 9$ 
C)  $y = - 8 x + 9$ 
D)  $y = - 9 x + 11$ 
E)  $y = - 4 x + 9$ 
A)  $y = - 11 x + 11$ 
B)  $y = - 2 x + 9$ 
C)  $y = - 8 x + 9$ 
D)  $y = - 9 x + 11$ 
E)  $y = - 4 x + 9$

Question 8

$\text { Find the derivative of the function } y = 8 \cos 4 x \text {. }$

Accepted Answer

A)  $y ^ { t} = - 4 \sin 4 x$ 
B)  $y ^ { t } = - 8 \sin 4 x$ 
C)  $y ^ { t } = - 32 \sin 4 x$ 
D)  $y ^ { t } = 32 \cos 4 x$ 
E)  $y ^ { t} = - 32 \cos 4 x$ 
A)  $y ^ { t} = - 4 \sin 4 x$ 
B)  $y ^ { t } = - 8 \sin 4 x$ 
C)  $y ^ { t } = - 32 \sin 4 x$ 
D)  $y ^ { t } = 32 \cos 4 x$ 
E)  $y ^ { t} = - 32 \cos 4 x$

Question 9

$\text { Use the rules of differentiation to find the derivative of the function } g ( x ) = x ^ { 6 } + 5 x ^ { 3 } \text {. }$

Accepted Answer

A)  $g ^ {t } ( x ) = 6 x ^ { 5 } + 3 x ^ { 2 }$ 
B)  $g ^ { t } ( x ) = x ^ { 5 } + 5 x ^ { 2 }$ 
C)  $g ^ { t} ( x ) = 6 x ^ { 5 } + 15 x ^ { 2 }$ 
D)  $g ^ { t } ( x ) = 6 x ^ { 7 } + 15 x ^ { 4 }$ 
E)  $g ^ { t } ( x ) = x ^ { 7 } + 5 x ^ { 4 }$ 
A)  $g ^ {t } ( x ) = 6 x ^ { 5 } + 3 x ^ { 2 }$ 
B)  $g ^ { t } ( x ) = x ^ { 5 } + 5 x ^ { 2 }$ 
C)  $g ^ { t} ( x ) = 6 x ^ { 5 } + 15 x ^ { 2 }$ 
D)  $g ^ { t } ( x ) = 6 x ^ { 7 } + 15 x ^ { 4 }$ 
E)  $g ^ { t } ( x ) = x ^ { 7 } + 5 x ^ { 4 }$

Question 10

$\text { Find an equation of the tangent line to the graph of the function } f ( x ) = \sqrt { x - 2 } \text { at }$ the point $( 18,4 )$.

Accepted Answer

A)  $y = \frac { x } { 4 } + \frac { 7 } { 2 }$ 
B)  $y = \frac { x } { 8 } + \frac { 7 } { 4 }$ 
C)  $y = \frac { x } { 8 } + \frac { 9 } { 2 }$ 
D)  $y = \frac { x } { 4 } + \frac { 9 } { 2 }$ 
E)  $y = \frac { x } { 8 } + \frac { 9 } { 4 }$ 
A)  $y = \frac { x } { 4 } + \frac { 7 } { 2 }$ 
B)  $y = \frac { x } { 8 } + \frac { 7 } { 4 }$ 
C)  $y = \frac { x } { 8 } + \frac { 9 } { 2 }$ 
D)  $y = \frac { x } { 4 } + \frac { 9 } { 2 }$ 
E)  $y = \frac { x } { 8 } + \frac { 9 } { 4 }$

Question 11

$\text { Find the second derivative of the function } f ( x ) = \frac { 3 x ^ { 2 } + 5 x - 4 } { x } \text {. }$

Accepted Answer

A)  $f ^ {tt } ( s ) = - \frac { 8 } { x ^ { 3 } }$ 
B)  $f ^ { tt } ( s ) = \frac { 8 } { x ^ { 3 } }$ 
C)  $f ^ {tt} ( s ) = - \frac { x + 8 } { x ^ { 3 } }$ 
D)  $f ^ { tt } ( s ) = \frac { 4 } { x ^ { 3 } }$ 
E)  $f ^ { tt } ( s ) = - \frac { 8 } { x ^ { 2 } }$ 
A)  $f ^ {tt } ( s ) = - \frac { 8 } { x ^ { 3 } }$ 
B)  $f ^ { tt } ( s ) = \frac { 8 } { x ^ { 3 } }$ 
C)  $f ^ {tt} ( s ) = - \frac { x + 8 } { x ^ { 3 } }$ 
D)  $f ^ { tt } ( s ) = \frac { 4 } { x ^ { 3 } }$ 
E)  $f ^ { tt } ( s ) = - \frac { 8 } { x ^ { 2 } }$

Question 12

$\text { Find the second derivative of the function } f ( x ) = \sin 5 x ^ { 6 } \text {. }$

Accepted Answer

A)  $f ^ { tt } ( x ) = 30 x ^ { 4 } \cos 5 x ^ { 6 } + 30 x ^ { 10 } \sin 5 x ^ { 6 }$ 
B)  $f ^ { tt } ( x ) = 30 x ^ { 4 } \cos 5 x ^ { 6 } - 900 x ^ { 10 } \sin 5 x ^ { 6 }$ 
C)  $f ^ { tt } ( x ) = 180 x ^ { 4 } \cos 5 x ^ { 6 } - 900 x ^ { 10 } \sin 5 x ^ { 6 }$ 
D)  $f ^ { tt } ( x ) = 150 x ^ { 4 } \cos 5 x ^ { 6 } + 900 x ^ { 10 } \sin 5 x ^ { 6 }$ 
E)  $f ^ { tt } ( x ) = 150 x ^ { 4 } \cos 5 x ^ { 6 } - 900 x ^ { 10 } \sin 5 x ^ { 6 }$ 
A)  $f ^ { tt } ( x ) = 30 x ^ { 4 } \cos 5 x ^ { 6 } + 30 x ^ { 10 } \sin 5 x ^ { 6 }$ 
B)  $f ^ { tt } ( x ) = 30 x ^ { 4 } \cos 5 x ^ { 6 } - 900 x ^ { 10 } \sin 5 x ^ { 6 }$ 
C)  $f ^ { tt } ( x ) = 180 x ^ { 4 } \cos 5 x ^ { 6 } - 900 x ^ { 10 } \sin 5 x ^ { 6 }$ 
D)  $f ^ { tt } ( x ) = 150 x ^ { 4 } \cos 5 x ^ { 6 } + 900 x ^ { 10 } \sin 5 x ^ { 6 }$ 
E)  $f ^ { tt } ( x ) = 150 x ^ { 4 } \cos 5 x ^ { 6 } - 900 x ^ { 10 } \sin 5 x ^ { 6 }$

Question 13

Find the derivative of the function. $$f ( t ) = \left( 4 + 7 t ^ { 5 } \right) ^ { 8 }$$

Accepted Answer

A)  $f ^ {t } ( t ) = 280 t ^ { 4 } \left( 4 + 7 t ^ { 4 } \right) ^ { 7 }$ 
B)  $f ^ {t } ( t ) = 280 t ^ { 4 } \left( 4 + 7 t ^ { 5 } \right) ^ { 7 }$ 
C)  $f ^ { t } ( t ) = 7 t ^ { 4 } \left( 4 + 7 t ^ { 5 } \right) ^ { 7 }$ 
D)  $f ^ { t } ( t ) = 280 t ^ { 7 } \left( 4 + 7 t ^ { 5 } \right) ^ { 7 }$ 
E)  $f ^ { t } ( t ) = 280 t ^ { 8 } \left( 4 + 7 t ^ { 5 } \right) ^ { 7 }$ 
A)  $f ^ {t } ( t ) = 280 t ^ { 4 } \left( 4 + 7 t ^ { 4 } \right) ^ { 7 }$ 
B)  $f ^ {t } ( t ) = 280 t ^ { 4 } \left( 4 + 7 t ^ { 5 } \right) ^ { 7 }$ 
C)  $f ^ { t } ( t ) = 7 t ^ { 4 } \left( 4 + 7 t ^ { 5 } \right) ^ { 7 }$ 
D)  $f ^ { t } ( t ) = 280 t ^ { 7 } \left( 4 + 7 t ^ { 5 } \right) ^ { 7 }$ 
E)  $f ^ { t } ( t ) = 280 t ^ { 8 } \left( 4 + 7 t ^ { 5 } \right) ^ { 7 }$

Question 14

$\text { Determine all values of } x \text {, (if any), at which the graph of the function has a }$ horizontal tangent.
$y ( x ) = \frac { 9 } { x - 6 }$

Accepted Answer

A)  $x = 9$ and $x = - 6$ 
B)  $x = 9$ 
C)  $x = 9$ and $x = 6$ 
D)  $x = 6$ 
E)  The graph has no horizontal tangents. 
A)  $x = 9$ and $x = - 6$ 
B)  $x = 9$ 
C)  $x = 9$ and $x = 6$ 
D)  $x = 6$ 
E)  The graph has no horizontal tangents.

Question 15

$\text { Find an equation of the tangent line to the graph of } y = e ^ { 3 x } \text { at the point } ( 0,1 ) \text {. }$

Accepted Answer

A)  $y = 3 x + 1$ 
B)  $y = x + 1$ 
C)  $y = 3 x - 1$ 
D)  $y = \ln ( 3 ) x + 1$ 
E)  $y = 4 x + 1$ 
A)  $y = 3 x + 1$ 
B)  $y = x + 1$ 
C)  $y = 3 x - 1$ 
D)  $y = \ln ( 3 ) x + 1$ 
E)  $y = 4 x + 1$

Question 16

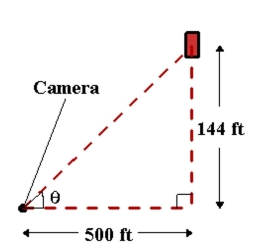
In a free-fall experiment, an object is dropped from a height of 144 feet. A camera on the ground 500 feet from the point of impact records the fall of the object as shown in the figure. Assuming the object is released at time $t = 0$. Find the rate of change of the angle of elevation of the camera when $t = 1$. Round your answer to four decimal places.

Accepted Answer

A)  $- 0.6006 \mathrm { rad } / \mathrm { sec }$ 
B)  $- 0.0581 \mathrm { rad } / \mathrm { sec }$ 
C)  $0.0601 \mathrm { rad } / \mathrm { sec }$ 
D)  $0.0581 \mathrm { rad } / \mathrm { sec }$ 
E)  $- 0.0601 \mathrm { rad } / \mathrm { sec }$ 
A)  $- 0.6006 \mathrm { rad } / \mathrm { sec }$ 
B)  $- 0.0581 \mathrm { rad } / \mathrm { sec }$ 
C)  $0.0601 \mathrm { rad } / \mathrm { sec }$ 
D)  $0.0581 \mathrm { rad } / \mathrm { sec }$ 
E)  $- 0.0601 \mathrm { rad } / \mathrm { sec }$

Question 17

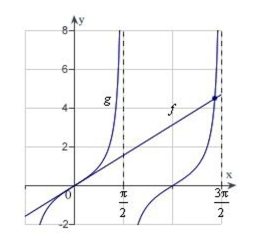
Apply Newton's Method to approximate the x-value of the indicated point of intersection of $f ( x ) = x \text { and } g ( x ) = \tan x \text { Continue the process until two successive approximations }$ differ by less than 0.001. (Hint: Let $h ( x ) = f ( x ) - g ( x )$.) Round your answer to three decimal places.

Accepted Answer

A)  $5.494$ 
B)  $8.725$ 
C)  $6.494$ 
D)  $7.725$ 
E)  $4.641$ 
A)  $5.494$ 
B)  $8.725$ 
C)  $6.494$ 
D)  $7.725$ 
E)  $4.641$

Question 18

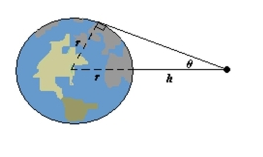
When satellites observe Earth, they can scan only part of Earth's surface. Some satellites have sensors that can measure the angle $\theta$ shown in the figure. Let $h$ represent the satellite's distance from Earth's surface and let $r$ represent Earth's radius. Find the rate at which $h$ is changing with respect to $\theta$ when $\theta = 60 ^ { \circ }$ (Assume $r = 4460$ miles.) Round your answer to the nearest unit.

Accepted Answer

A)  $- 2973 \mathrm { mi } / \mathrm { radian }$ 
B)  $- 5150 \mathrm { mi } / \mathrm { radian }$ 
C)  $5150 \mathrm { mi } / \mathrm { radian }$ 
D)  $- 8920 \mathrm { mi } / \mathrm { radian }$ 
E)  $2973 \mathrm { mi } / \mathrm { radian }$ 
A)  $- 2973 \mathrm { mi } / \mathrm { radian }$ 
B)  $- 5150 \mathrm { mi } / \mathrm { radian }$ 
C)  $5150 \mathrm { mi } / \mathrm { radian }$ 
D)  $- 8920 \mathrm { mi } / \mathrm { radian }$ 
E)  $2973 \mathrm { mi } / \mathrm { radian }$

Question 19

$\text { The length of a rectangle is } 5 t + 5 \text { and its height is } t ^ { 4 } \text {, where } t \text { is time in seconds and }$ the dimensions are in inches. Find the rate of change of area, A, with respect to time.

Accepted Answer

A)  $\frac { d A } { d t } = t ^ { 4 } ( 20 + 25 t )$ square inches/second 
B)  $\frac { d A } { d t } = t ^ { 4 } ( 15 + 25 t )$ square inches/second 
C)  $\frac { d A } { d t } = t ^ { 3 } ( 20 + 20 t )$ square inches/second 
D)  $\frac { d A } { d t } = t ^ { 3 } ( 20 + 25 t )$ square inches/second 
E)  $\frac { d A } { d t } = t ^ { 3 } ( 25 t + 5 )$ square inches/second 
A)  $\frac { d A } { d t } = t ^ { 4 } ( 20 + 25 t )$ square inches/second 
B)  $\frac { d A } { d t } = t ^ { 4 } ( 15 + 25 t )$ square inches/second 
C)  $\frac { d A } { d t } = t ^ { 3 } ( 20 + 20 t )$ square inches/second 
D)  $\frac { d A } { d t } = t ^ { 3 } ( 20 + 25 t )$ square inches/second 
E)  $\frac { d A } { d t } = t ^ { 3 } ( 25 t + 5 )$ square inches/second

Question 20

$\text { A point is moving along the graph of the function } y = \frac { 1 } { 9 x ^ { 2 } + 4 } \text { such that } \frac { d x } { d t } = 2$ centimeters per second. Find $\frac { d y } { d t }$ when $x = 2$.

Accepted Answer

A)  $\frac { d y } { d t } = - \frac { 9 } { 5 }$ 
B)  $\frac { d y } { d t } = \frac { 9 } { 200 }$ 
C)  $\frac { d y } { d t } = \frac { 9 } { 400 }$ 
D)  $\frac { d y } { d t } = - \frac { 9 } { 400 }$ 
E)  $\frac { d y } { d t } = - \frac { 9 } { 200 }$ 
A)  $\frac { d y } { d t } = - \frac { 9 } { 5 }$ 
B)  $\frac { d y } { d t } = \frac { 9 } { 200 }$ 
C)  $\frac { d y } { d t } = \frac { 9 } { 400 }$ 
D)  $\frac { d y } { d t } = - \frac { 9 } { 400 }$ 
E)  $\frac { d y } { d t } = - \frac { 9 } { 200 }$

$\text { Find } \frac { d y } { d x } \text { by implicit differentiation. }$ $x ^ { \frac { 6 } { 7 } } + y ^ { \frac { 8 } { 5 } } = 9$

Find the derivative of the function. $f ( t ) = ( 1 + 8 t ) ^ { \frac { 5 } { 9 } }$

Find the points at which the graph of the equation has a vertical or horizontal tangent line. $5 x ^ { 2 } + 4 y ^ { 2 } - 10 x + 24 y + 8 = 0$

The radius r of a sphere is increasing at a rate of inches per minute. Find the rate of change of the volume when $r = 11 \text { inches. }$

$\text { Find the derivative of the function } y = \ln \left( x \sqrt { x ^ { 2 } + 7 } \right) \text {. }$

$\text { Evaluate the derivative of the function } f ( t ) = \frac { 8 t ^ { 2 } + 2 } { 5 t - 1 } \text { at the point } \left( 4 , \frac { 130 } { 19 } \right) \text {. }$

Use implicit differentiation to find an equation of the tangent line to the ellipse $\frac { x ^ { 2 } } { 2 } + \frac { y ^ { 2 } } { 98 } = 1 \text { at } ( 1,7 )$

$\text { Find the derivative of the function } y = 8 \cos 4 x \text {. }$

$\text { Use the rules of differentiation to find the derivative of the function } g ( x ) = x ^ { 6 } + 5 x ^ { 3 } \text {. }$

$\text { Find an equation of the tangent line to the graph of the function } f ( x ) = \sqrt { x - 2 } \text { at }$ the point $( 18,4 )$ .

$\text { Find the second derivative of the function } f ( x ) = \frac { 3 x ^ { 2 } + 5 x - 4 } { x } \text {. }$

$\text { Find the second derivative of the function } f ( x ) = \sin 5 x ^ { 6 } \text {. }$

Find the derivative of the function. $f ( t ) = \left( 4 + 7 t ^ { 5 } \right) ^ { 8 }$

$\text { Determine all values of } x \text {, (if any), at which the graph of the function has a }$ horizontal tangent. $y ( x ) = \frac { 9 } { x - 6 }$

$\text { Find an equation of the tangent line to the graph of } y = e ^ { 3 x } \text { at the point } ( 0,1 ) \text {. }$

$\text { The length of a rectangle is } 5 t + 5 \text { and its height is } t ^ { 4 } \text {, where } t \text { is time in seconds and }$ the dimensions are in inches. Find the rate of change of area, A, with respect to time.

$\text { A point is moving along the graph of the function } y = \frac { 1 } { 9 x ^ { 2 } + 4 } \text { such that } \frac { d x } { d t } = 2$ centimeters per second. Find $\frac { d y } { d t }$ when $x = 2$ .

Graphs and Models

A Preview of Calculus

Extrema on an Interval

Antiderivatives and Indefinite Integration

Slope Fields and Eulers Method

Area of a Region Between Two Curves

Basic Integration Rules

Sequences

Conics and Calculus

Vectors in the Plane

Vector-Valued Functions

Introduction to Functions of Several Variables

Iterated Integrals and Area in the Plane

Vector Fields

Exact First-Order Equations

Filters

Exam 3: The Derivative and the Tangent Line Problem

Find dydx by implicit differentiation. \text { Find } \frac { d y } { d x } \text { by implicit differentiation. } Find dxdy​ by implicit differentiation. x67+y85=9x ^ { \frac { 6 } { 7 } } + y ^ { \frac { 8 } { 5 } } = 9x76​+y58​=9

Find the derivative of the function. f(t)=(1+8t)59f ( t ) = ( 1 + 8 t ) ^ { \frac { 5 } { 9 } }f(t)=(1+8t)95​

Find the points at which the graph of the equation has a vertical or horizontal tangent line. 5x2+4y2−10x+24y+8=05 x ^ { 2 } + 4 y ^ { 2 } - 10 x + 24 y + 8 = 05x2+4y2−10x+24y+8=0

The radius r of a sphere is increasing at a rate of inches per minute. Find the rate of change of the volume when r=11 inches. r = 11 \text { inches. }r=11 inches.

Find the derivative of the function y=ln⁡(xx2+7). \text { Find the derivative of the function } y = \ln \left( x \sqrt { x ^ { 2 } + 7 } \right) \text {. } Find the derivative of the function y=ln(xx2+7​).

Use implicit differentiation to find an equation of the tangent line to the ellipse x22+y298=1 at (1,7)\frac { x ^ { 2 } } { 2 } + \frac { y ^ { 2 } } { 98 } = 1 \text { at } ( 1,7 )2x2​+98y2​=1 at (1,7)

Find the derivative of the function y=8cos⁡4x. \text { Find the derivative of the function } y = 8 \cos 4 x \text {. } Find the derivative of the function y=8cos4x.

Use the rules of differentiation to find the derivative of the function g(x)=x6+5x3. \text { Use the rules of differentiation to find the derivative of the function } g ( x ) = x ^ { 6 } + 5 x ^ { 3 } \text {. } Use the rules of differentiation to find the derivative of the function g(x)=x6+5x3.

Find the second derivative of the function f(x)=3x2+5x−4x. \text { Find the second derivative of the function } f ( x ) = \frac { 3 x ^ { 2 } + 5 x - 4 } { x } \text {. } Find the second derivative of the function f(x)=x3x2+5x−4​.

Find the second derivative of the function f(x)=sin⁡5x6. \text { Find the second derivative of the function } f ( x ) = \sin 5 x ^ { 6 } \text {. } Find the second derivative of the function f(x)=sin5x6.

Find the derivative of the function. f(t)=(4+7t5)8f ( t ) = \left( 4 + 7 t ^ { 5 } \right) ^ { 8 }f(t)=(4+7t5)8

Find an equation of the tangent line to the graph of y=e3x at the point (0,1). \text { Find an equation of the tangent line to the graph of } y = e ^ { 3 x } \text { at the point } ( 0,1 ) \text {. } Find an equation of the tangent line to the graph of y=e3x at the point (0,1).

Graphs and Models

A Preview of Calculus

Extrema on an Interval

Antiderivatives and Indefinite Integration

Slope Fields and Eulers Method

Area of a Region Between Two Curves

Basic Integration Rules

Sequences

Conics and Calculus

Vectors in the Plane

Vector-Valued Functions

Introduction to Functions of Several Variables

Iterated Integrals and Area in the Plane

Vector Fields

Exact First-Order Equations

Filters

$\text { Find } \frac { d y } { d x } \text { by implicit differentiation. }$ $x ^ { \frac { 6 } { 7 } } + y ^ { \frac { 8 } { 5 } } = 9$

Find the derivative of the function. $f ( t ) = ( 1 + 8 t ) ^ { \frac { 5 } { 9 } }$

Find the points at which the graph of the equation has a vertical or horizontal tangent line. $5 x ^ { 2 } + 4 y ^ { 2 } - 10 x + 24 y + 8 = 0$

The radius r of a sphere is increasing at a rate of inches per minute. Find the rate of change of the volume when $r = 11 \text { inches. }$

$\text { Find the derivative of the function } y = \ln \left( x \sqrt { x ^ { 2 } + 7 } \right) \text {. }$

Use implicit differentiation to find an equation of the tangent line to the ellipse $\frac { x ^ { 2 } } { 2 } + \frac { y ^ { 2 } } { 98 } = 1 \text { at } ( 1,7 )$

$\text { Find the derivative of the function } y = 8 \cos 4 x \text {. }$

$\text { Use the rules of differentiation to find the derivative of the function } g ( x ) = x ^ { 6 } + 5 x ^ { 3 } \text {. }$

$\text { Find the second derivative of the function } f ( x ) = \frac { 3 x ^ { 2 } + 5 x - 4 } { x } \text {. }$

$\text { Find the second derivative of the function } f ( x ) = \sin 5 x ^ { 6 } \text {. }$

Find the derivative of the function. $f ( t ) = \left( 4 + 7 t ^ { 5 } \right) ^ { 8 }$

$\text { Find an equation of the tangent line to the graph of } y = e ^ { 3 x } \text { at the point } ( 0,1 ) \text {. }$