Exam 3: The Derivative and the Tangent Line Problem

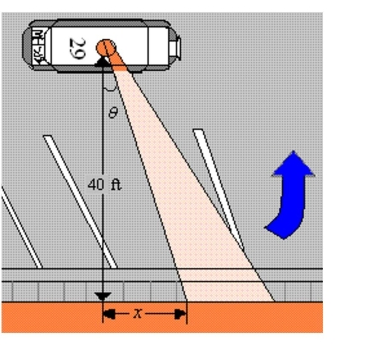
A petrol car is parked 40 feet from a long warehouse (see figure). The revolving light on top $\text { of the car turns at a rate of } 30 \text { revolutions per minute. Write } \theta \text { as a function of } x \text {. }$

Find an equation to the tangent line for the graph of f at the given point. $f ( x ) = \left( 5 x ^ { 5 } + 5 \right) ^ { 2 } , \quad ( 1,100 )$

Find the derivative of the function. $g ( x ) = \left( \frac { x + 6 } { x ^ { 2 } + 7 } \right) ^ { 4 }$

$\text { Find the derivative of the algebraic function } f ( x ) = \left( x ^ { 6 } + 4 \right) ^ { 5 } \text {. }$

$\text { Find } f ^ { t } ( x ) \text { if } f ( x ) = \log _ { 3 } \left( \frac { x ^ { 2 } - 5 } { x - 2 } \right)$

$\frac { d y } { d x } \text { at the point } ( 0,20 ) \text { for the equation } x = 14 \ln \left( y ^ { 2 } - 399 \right) \text {. }$

Find the derivative of the function. $f ( s ) = 9 s \sin s + 5 \cos s .$

Find an equation of the tangent line to the graph of f at the given point. $f ( t ) = ( t - 5 ) \left( t ^ { 2 } - 3 \right) , \text { at } ( 2 , - 3 )$

$\text { A buoy oscillates in simple harmonic motion } y = A \cos \text { at as waves move past }$ it. The buoy moves a total of $13.5$ feet (vertically) between its low point and its high point. It returns to its high point every 10 seconds. Determine the velocity of the buoy as a function of $t$ .

Find the slope-intercept form of the equation of the line tangent to the graph of $y = \arctan ( 7 x )$ when $x = \frac { \sqrt { 3 } } { 7 }$

$\text { Find } \frac { d ^ { 2 } y } { d ^ { 2 } x } \text { in terms of } x \text { and } y \text { given that } x ^ { 2 } + 6 y ^ { 2 } = 9 \text {. Use the original equation to }$ simplify your answer.

Determine all values of x, (if any), at which the graph of the function has a horizontal tangent. $y ( x ) = \frac { 6 x } { ( x - 9 ) ^ { 2 } }$

Find the slope of the graph of the function at the given value. $f ( x ) = \frac { - 5 } { x ^ { 3 } }$ when $x = 9$

Complete two iterations of Newton's Method for the function $f ( x ) = x ^ { 2 } - 7 \text { using the }$ initial guess $\text { 2.6. Round your answers to four decimal places. }$

$\text { The graph of the function } f \text { is given below. Select the graph of } f ^ { t } \text {. }$

$\text { Use logarithmic differentiation to find the derivative of } y = \frac { x ^ { 36 } \sqrt { 29 x - 4 } } { ( x - 1 ) ^ { 14 } } \text {. }$

airplane is flying in still air with an airspeed of miles per hour. If it is climbing at an angle of , find the rate at which it is gaining altitude. Round your answer to four Decimal places.

$\text { Find the derivative of the algebraic function } f ( x ) = x \left( 3 - \frac { 4 } { x + 6 } \right) \text {. }$

$\text { Find the derivative of the function } g ( x ) = - 2 \text { by the limit process. }$

$\text { Use the rules of differentiation to find the derivative of the function } y = 7 + 6 \sin x \text {. }$