Exam 14: Iterated Integrals and Area in the Plane
Exam 1: Graphs and Models114 Questions
Exam 2: A Preview of Calculus92 Questions
Exam 3: The Derivative and the Tangent Line Problem191 Questions
Exam 4: Extrema on an Interval147 Questions
Exam 5: Antiderivatives and Indefinite Integration167 Questions
Exam 6: Slope Fields and Eulers Method85 Questions
Exam 7: Area of a Region Between Two Curves120 Questions
Exam 8: Basic Integration Rules127 Questions
Exam 9: Sequences179 Questions
Exam 10: Conics and Calculus120 Questions
Exam 11: Vectors in the Plane125 Questions
Exam 12: Vector-Valued Functions83 Questions
Exam 13: Introduction to Functions of Several Variables124 Questions
Exam 14: Iterated Integrals and Area in the Plane118 Questions
Exam 15: Vector Fields108 Questions
Exam 16: Exact First-Order Equations45 Questions
Select questions type
Find the mass and center of mass of the lamina bounded by the graphs of the equations given below for the given density.
(Multiple Choice)
4.9/5  (34)
(34)
Use a double integral in polar coordinates to find the volume of the solid in the first octant bounded by the graphs of the equations given below.
(Multiple Choice)
4.9/5  (31)
(31)
Find the area of the surface of the portion of the plane
in the first octant.
(Multiple Choice)
4.9/5  (37)
(37)
Use a triple integral to find the volume of the solid shown below. 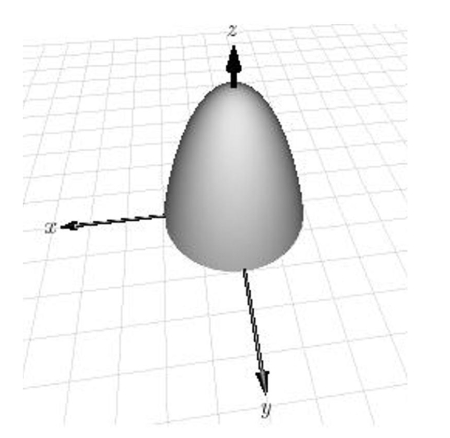
(Multiple Choice)
4.8/5  (28)
(28)
Use spherical coordinates to find the z coordinate of the center of mass of the solid lying between two concentric hemispheres of radii 6 and 7, and having uniform density k.
(Multiple Choice)
4.9/5  (39)
(39)
Sketch the image S in the uv-plane of the region R in the xy-plane using the given transformation.
x=2u+3v y=2v
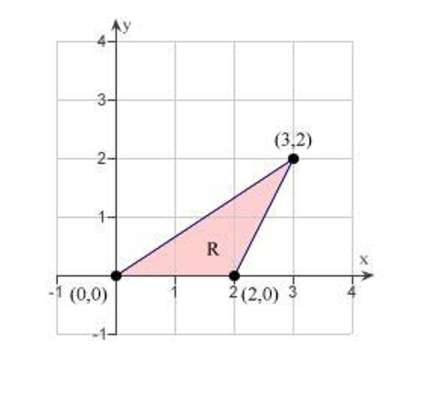
(Multiple Choice)
4.9/5  (35)
(35)
Determine the location of the horizontal axis gate in a dam is to be hinged so that there is no moment causing rotation under the indicated loading (see figure (a)). The model for is where is the -coordinate of the centroid of the gate, is the moment of inertia of the gate about the line is the depth of the centroid below the surface, and is the area of the gate.

(Multiple Choice)
4.8/5  (32)
(32)
Set up a triple integral that gives the moment of inertia about the region of density given below.
\rho(x,y,z)= Q=\{-4\leqx\leq4,-4\leqy\leq4,0\leqz\leq1-y\}
(Multiple Choice)
4.8/5  (33)
(33)
Suppose the temperature in degrees Celsius on the surface of a metal plate is , where and are measured in centimeters. Estimate the average temperature if varies between 0 and 2 centimeters and varies between 0 and 7 centimeters.
(Multiple Choice)
4.7/5  (21)
(21)
Find the Jacobian for the change of variables given below.
(Multiple Choice)
4.9/5  (36)
(36)
Use polar coordinates to describe the region as shown in the figure below: 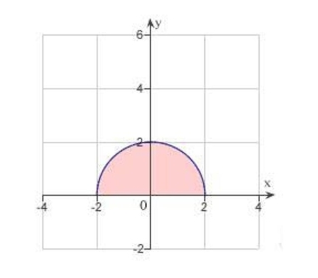
(Multiple Choice)
4.8/5  (36)
(36)
Use an iterated integral to find the area of the region bounded by
(Multiple Choice)
4.9/5  (34)
(34)
Set up and evaluate a double integral required to find the moment of inertia, I, about the given line, of the lamina bounded by the graphs of the following equations. Use a computer Algebra system to evaluate the double integral. , line:
(Multiple Choice)
4.9/5  (41)
(41)
Find the area of the portion of the surface the region Round your answer to two decimal places.
(Multiple Choice)
4.8/5  (34)
(34)
Combine the sum of the two iterated integrals into a single integral by converting to polar coordinates. Evaluate the resulting iterated integral.
(Multiple Choice)
4.7/5  (26)
(26)
Find the center of mass of the lamina bounded by the graphs of the equations , and for the density
(Multiple Choice)
4.9/5  (29)
(29)
Showing 101 - 118 of 118
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)