Exam 16: Integrating Functions of Several Variables
Exam 1: A Library of Functions110 Questions
Exam 2: Key Concept: the Derivative92 Questions
Exam 3: Short-Cuts to Differentiation175 Questions
Exam 4: Using the Derivative108 Questions
Exam 5: Key Concept- the Definite Integral62 Questions
Exam 6: Constructing Antiderivatives90 Questions
Exam 7: Integration179 Questions
Exam 8: Using the Definite Integral104 Questions
Exam 9: Sequences and Series70 Questions
Exam 10: Approximating Functions Using Series71 Questions
Exam 11: Differential Equations135 Questions
Exam 12: Functions of Several Variables93 Questions
Exam 13: A Fundamental Tool- Vectors107 Questions
Exam 14: Differentiating Functions of Several Variables129 Questions
Exam 15: Optimization- Local and Global Extrema77 Questions
Exam 16: Integrating Functions of Several Variables76 Questions
Exam 17: Parameterization and Vector Fields86 Questions
Exam 18: Line Integrals78 Questions
Exam 19: Flux Integrals and Divergence52 Questions
Exam 20: The Curl and Stokes Theorem84 Questions
Exam 21: Parameters, Coordinates, Integrals23 Questions
Select questions type
Find a region R such that double integral has the largest value.
(Multiple Choice)
4.9/5  (29)
(29)
Consider the change of variables x = s + 5t, y = s - 3t.
Find the absolute value of the Jacobian .
(Short Answer)
4.9/5  (32)
(32)
Let W be the region between the cylinders and in the first octant and under the plane z = 1.Evaluate
(Essay)
4.8/5  (24)
(24)
Set up but do not evaluate a (multiple)integral that gives the volume of the solid bounded above by the sphere and below by the paraboloid .
(Multiple Choice)
4.8/5  (47)
(47)
Evaluate the integral by interchanging the order of integration. .
(Short Answer)
4.7/5  (31)
(31)
Rewrite the integral in spherical coordinates.You do not have to evaluate the integral.
(Essay)
5.0/5  (31)
(31)
For the following region, decide whether to integrate using polar or Cartesian coordinates.Write an iterated integral of an arbitrary function f(x, y)over the region. 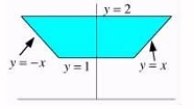
(Essay)
4.9/5  (35)
(35)
Evaluate the integral , where R is the region in the first quadrant bounded by the y-axis, the line y = x and the circles
(Essay)
4.7/5  (37)
(37)
Convert the integral to polar coordinates and hence evaluate it exactly.
(Essay)
4.8/5  (36)
(36)
Let W be the part of the solid sphere of radius 4, centered at the origin, that lies above the plane z = 2.Express in
(a)Cartesian
(b)Cylindrical
(c)Spherical coordinates.
(Essay)
4.9/5  (37)
(37)
The joint density function for x, y is given by Write down an iterated integral to compute the probability that x + y 10.You do not need to do the integral.
(Multiple Choice)
4.9/5  (34)
(34)
Let R1 be the region 0 x 3, -2 y 4, and let R2 be the region 3 x 5, -2 y 4.Suppose that the average value of f over R1 is 6 and the average value over R2 is 7.
Find the average value of f over R, .
(Short Answer)
4.9/5  (33)
(33)
Consider the integral Rewrite the integral with the integration performed in the opposite order.
(Multiple Choice)
4.9/5  (41)
(41)
Find the area of the part of the hyperbolic paraboloid that lies between the cylinders and .
(Multiple Choice)
4.9/5  (39)
(39)
Find the condition on the non-negative constants a and b for p(x, y)to be a joint density function, where
(Multiple Choice)
4.8/5  (38)
(38)
Sketch the region of integration of the following integral and then convert the expression to polar co-ordinates (you do not have to evaluate it).
(Essay)
4.8/5  (41)
(41)
Calculate the following integral exactly.(Your answer should not be a decimal approximating the true answer, but should be exactly equal to the true answer.Your answer may contain e, , , and so on.)
(Essay)
4.8/5  (42)
(42)
Showing 41 - 60 of 76
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)