Exam 14: Differentiating Functions of Several Variables
Exam 1: A Library of Functions110 Questions
Exam 2: Key Concept: the Derivative92 Questions
Exam 3: Short-Cuts to Differentiation175 Questions
Exam 4: Using the Derivative108 Questions
Exam 5: Key Concept- the Definite Integral62 Questions
Exam 6: Constructing Antiderivatives90 Questions
Exam 7: Integration179 Questions
Exam 8: Using the Definite Integral104 Questions
Exam 9: Sequences and Series70 Questions
Exam 10: Approximating Functions Using Series71 Questions
Exam 11: Differential Equations135 Questions
Exam 12: Functions of Several Variables93 Questions
Exam 13: A Fundamental Tool- Vectors107 Questions
Exam 14: Differentiating Functions of Several Variables129 Questions
Exam 15: Optimization- Local and Global Extrema77 Questions
Exam 16: Integrating Functions of Several Variables76 Questions
Exam 17: Parameterization and Vector Fields86 Questions
Exam 18: Line Integrals78 Questions
Exam 19: Flux Integrals and Divergence52 Questions
Exam 20: The Curl and Stokes Theorem84 Questions
Exam 21: Parameters, Coordinates, Integrals23 Questions
Select questions type
If fx(0, 0)exists and fy(0, 0)exists, then f is differentiable at (0, 0).
(True/False)
4.9/5  (40)
(40)
Consider the function (a)Describe the level set g = 16.
(b)Find a vector perpendicular to the tangent plane to the level set g = 16 at the point (-1, 2, 2).
(Essay)
4.8/5  (28)
(28)
If is a unit vector and the level curves of f(x, y)are given below, then at point P we have 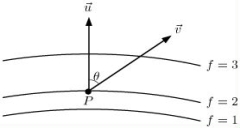
(True/False)
4.9/5  (27)
(27)
Consider the function (a)Find gx(x, y)and gy(x, y)for (x, y) (0, 0).
(b)Use the limit definition of partial derivative to show that gx(0, 0)= 0 and gy(0, 0)= 0.
(c)Are the functions gx and gy continuous at (0, 0)? Explain.
(d)Is g differentiable at (0, 0)? Explain.
(Essay)
4.7/5  (30)
(30)
Given that f(2, 4)= 1.5 and f(2.1, 4.4)= 2.1, estimate the value of , where is the unit vector in the direction of Give your answer to four decimal places.
(Essay)
4.8/5  (37)
(37)
The figure below shows the graph of z = f(x, y)and its intersection with various planes.(The x and y-axes have the same scale.) What is the sign of ? 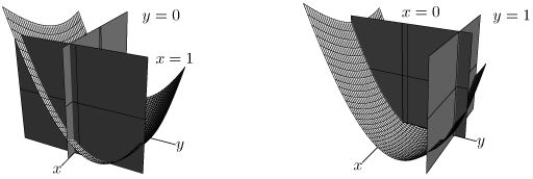
(Multiple Choice)
4.9/5  (47)
(47)
Using the contour diagram for f(x, y), find the sign of given that fxx(P)< 0. 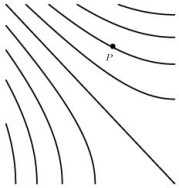
(Multiple Choice)
4.7/5  (27)
(27)
Find an equation for the tangent plane to the ellipsoid at the point .
(Essay)
5.0/5  (31)
(31)
Suppose that the function and the linear function satisfy for points close to .Find and .
(Essay)
4.8/5  (44)
(44)
Consider the surface and the point .Find a vector normal to the surface at P.
(Essay)
4.7/5  (29)
(29)
The table below gives values of a function f(x, y)near x = 1, y = 2. 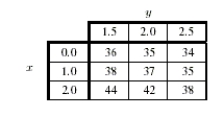 Give the equation of the tangent plane to the graph z = f(x, y)at x = 1, y = 2.
Give the equation of the tangent plane to the graph z = f(x, y)at x = 1, y = 2.
(Multiple Choice)
4.7/5  (29)
(29)
Showing 81 - 100 of 129
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)