Exam 4: Using the Derivative

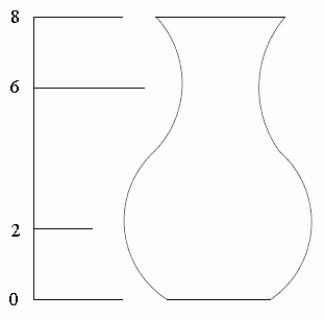
Starting at time t = 0, water is poured at a constant rate into an empty vase (pictured below).It takes ten seconds for the vase to be filled completely to the top.Let h = f(t)be the depth of the water in the vase at time t.For what value of h is $f^{\prime}(t)$ the largest? $Starting at time t = 0, water is poured at a constant rate into an empty vase (pictured below).It takes ten seconds for the vase to be filled completely to the top.Let h = f(t)be the depth of the water in the vase at time t.For what value of h is f^{\prime}(t) the largest?$

(Short Answer)

4.7/5

(34)

Question 41

Given the function $f(x)=x^{2} e^{-2 x}$ , then at x = 1 the graph of f has

(Multiple Choice)

4.9/5

(37)

Question 42

A rectangular swimming pool is 10 meters long and 6 meters wide.It has a depth of 1 meter at the shallow end, then slopes to a depth of 1.5 meters at the deep end, as shown in the following cross section (not to scale).It is being filled with a hose at a rate of 50,000 cubic centimeters per minute.225 minutes after the hose is turned on, the water is rising at a rate of _____ cm per second.Round to 3 decimal places.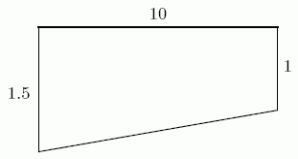

(Short Answer)

4.8/5

(33)

Question 43

Sketch a graph of a function whose $f^{\prime}(x)=0$ at x=1, $f^{\prime}(x)$ < 0 when x< 1, $f^{\prime}(x)$ < 0 when x> 1, Is it possible to have $f^{\prime \prime}(x)=0$ at any value from -2<x<2?

(Short Answer)

4.9/5

(40)

Question 44

Motion of a particle is given by $x=t^{2}-3 t, \quad y=2 t^{3}-3 t$ where t is time in minutes.Find the instantaneous speed of the particle at t = 3.Round to 2 decimal places.

(Short Answer)

4.8/5

(24)

Question 45

Consider the function $f(x)=a x(1-x)$ for $a>0$ .What is largest value of a such that $f(x) \leq 3$ on the region $0 \leq x \leq 1$ ?

(Short Answer)

4.7/5

(44)

Question 46

Graph the function $f(x)=x^{2} e^{-x}$ for $-1 \leq x \leq 3$ .

(Essay)

4.8/5

(32)

Question 47

A bar of ice cream, with dimensions of 3 cm by 3 cm by 3 cm placed on a mesh screen on top of a cylindrical funnel that is 6 cm high and 6 cm in diameter.If the ice cream is melting at a rate of 3.3 $\mathrm{cm}^{3} / \mathrm{min}$ into the funnel, what is the rate of change of the height of the funnel when half of the ice cream has melted? $V_{f u m a l}=\frac{1}{3} \pi r^{2} h$

(Multiple Choice)

4.8/5

(32)

Question 48

A normal distribution in statistics is modeled by the function $N(x)=\frac{1}{\sqrt{2 \pi}} e^{-x^{2} / 2}$ determine the limit of N(x)as $x \rightarrow$ - $\infty$

(Multiple Choice)

4.8/5

(33)

Question 49

In the function y=2 sin (x)+1.96, in the interval from 0 $\leq x \leq$ $\pi$ , at which value(s)of x does the function contain a global maximum?

(Multiple Choice)

4.9/5

(30)

Question 50

Below is the graph of the rate r at which people arrive for lunch at Cafeteria Charlotte.Checkers start at 12:00 noon and can pass people through at a constant rate of 5 people/minute.Let f(t)be the length of the line (i.e.the number of people)at time t.Suppose that at 11:50 there are already 150 people lined up.Using the graph together with this information, is the time 12:34 a local minimum, a local maximum, or neither of f?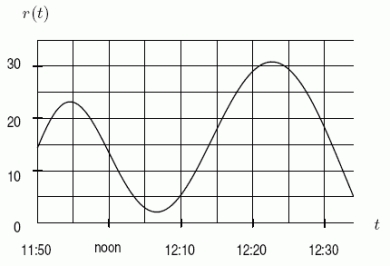

(Short Answer)

4.9/5

(36)

Question 51

Air is being blown into a spherical balloon at a rate of 70 cm³ per second.At what rate is the surface area of the balloon increasing when the radius is 10 cm? Round to 2 decimal places, and do not include units.

(Short Answer)

4.8/5

(28)

Question 52

A water tank is constructed in the shape of a sphere seated atop a circular cylinder.If water is being pumped into the tank at a constant rate, let $h(t)$ be the height of the water as a function of time.For the interval a < t < b, which of the following is true?

(Multiple Choice)

4.7/5

(28)

Question 53

Consider a continuous function with the following properties: $Consider a continuous function with the following properties: f(0)=3 \left|f^{\prime}(x)\right|<0.5 f^{\prime \prime}(x)<0 for x<0 f^{\prime}(1)=0 . Which of the following are inconsistent with these four conditions?$ $f(0)=3$ $Consider a continuous function with the following properties: f(0)=3 \left|f^{\prime}(x)\right|<0.5 f^{\prime \prime}(x)<0 for x<0 f^{\prime}(1)=0 . Which of the following are inconsistent with these four conditions?$ $\left|f^{\prime}(x)\right|<0.5$ $Consider a continuous function with the following properties: f(0)=3 \left|f^{\prime}(x)\right|<0.5 f^{\prime \prime}(x)<0 for x<0 f^{\prime}(1)=0 . Which of the following are inconsistent with these four conditions?$ $f^{\prime \prime}(x)<0$ for $x<0$ $Consider a continuous function with the following properties: f(0)=3 \left|f^{\prime}(x)\right|<0.5 f^{\prime \prime}(x)<0 for x<0 f^{\prime}(1)=0 . Which of the following are inconsistent with these four conditions?$ $f^{\prime}(1)=0$ . Which of the following are inconsistent with these four conditions?

(Multiple Choice)

4.9/5

(33)

Question 54

Let $f(x)=e^{\frac{-x^{2}}{b}}$ , where b is a positive constant.Which of the following are inflection points of f?

(Multiple Choice)

4.7/5

(33)

Question 55

A submarine can travel 30mi/hr submerged and 60mi/hr on the surface.The submarine must stay submerged if within 200 miles of shore.Suppose that this submarine wants to meet a surface ship 200 miles off shore.The submarine leaves from a port 300 miles along the coast from the surface ship.What route of the type sketched below should the sub take to minimize its time to rendezvous? Give the value of y to 2 decimal places.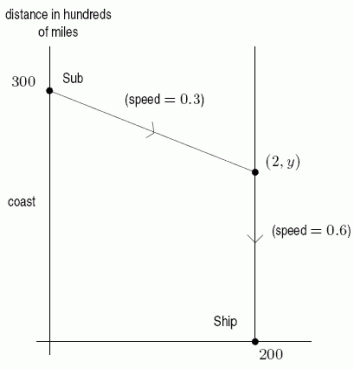

(Short Answer)

4.8/5

(42)

Question 56

A cupful of olive oil falls on the floor forming a circular puddle.Its radius is increasing at a constant rate of 0.2 cm/sec.What is the rate of increase in the area of the olive oil when its circumference measures 20 $\pi$ cm?

(Essay)

4.8/5

(39)

Question 57

One fine day you take a hike up a mountain path.Using your trusty map you have determined that the path is approximately in the shape of the curve $y=3\left(x^{3}-12 x^{2}+48 x+36\right)$ .Here y is the elevation in feet above sea level and x is the horizontal distance in miles you have traveled, but your map only shows the path for 7 miles, horizontal distance.How many feet above sea level are you when you start your hike?

(Short Answer)

4.8/5

(35)

Question 58

A single cell of a bee's honey comb has the shape shown.The surface area of this cell is given by $A=6 h s+\frac{3}{2} s^{2}\left(\frac{-\cos \theta}{\sin \theta}+\frac{\sqrt{3}}{\sin \theta}\right)$ where h, s, $\theta$ are as shown in the picture.Keeping h and s fixed, for what angle, $\theta$ , is the surface area minimal? Round to the nearest one tenth of a degree. $A single cell of a bee's honey comb has the shape shown.The surface area of this cell is given by A=6 h s+\frac{3}{2} s^{2}\left(\frac{-\cos \theta}{\sin \theta}+\frac{\sqrt{3}}{\sin \theta}\right) where h, s, \theta are as shown in the picture.Keeping h and s fixed, for what angle, \theta , is the surface area minimal? Round to the nearest one tenth of a degree.$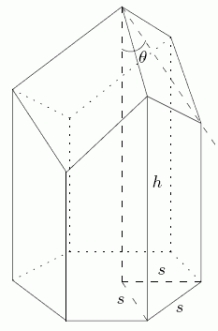

(Short Answer)

4.9/5

(42)

Question 59

Consider the two-parameter family of curves $y=\alpha x+\frac{b}{x}$ , with $a>0$ and $b>0$ .Is the graph concave up or down at the point x = -2?

(Multiple Choice)

4.8/5

(25)

Question 60

Showing 41 - 60 of 108

Given the function $f(x)=x^{2} e^{-2 x}$ , then at x = 1 the graph of f has

Sketch a graph of a function whose $f^{\prime}(x)=0$ at x=1, $f^{\prime}(x)$ < 0 when x< 1, $f^{\prime}(x)$ < 0 when x> 1, Is it possible to have $f^{\prime \prime}(x)=0$ at any value from -2<x<2?

Motion of a particle is given by $x=t^{2}-3 t, \quad y=2 t^{3}-3 t$ where t is time in minutes.Find the instantaneous speed of the particle at t = 3.Round to 2 decimal places.

Consider the function $f(x)=a x(1-x)$ for $a>0$ .What is largest value of a such that $f(x) \leq 3$ on the region $0 \leq x \leq 1$ ?

Graph the function $f(x)=x^{2} e^{-x}$ for $-1 \leq x \leq 3$ .

A normal distribution in statistics is modeled by the function $N(x)=\frac{1}{\sqrt{2 \pi}} e^{-x^{2} / 2}$ determine the limit of N(x)as $x \rightarrow$ - $\infty$

In the function y=2 sin (x)+1.96, in the interval from 0 $\leq x \leq$ $\pi$ , at which value(s)of x does the function contain a global maximum?

Air is being blown into a spherical balloon at a rate of 70 cm³ per second.At what rate is the surface area of the balloon increasing when the radius is 10 cm? Round to 2 decimal places, and do not include units.

A water tank is constructed in the shape of a sphere seated atop a circular cylinder.If water is being pumped into the tank at a constant rate, let $h(t)$ be the height of the water as a function of time.For the interval a < t < b, which of the following is true?

Let $f(x)=e^{\frac{-x^{2}}{b}}$ , where b is a positive constant.Which of the following are inflection points of f?

A cupful of olive oil falls on the floor forming a circular puddle.Its radius is increasing at a constant rate of 0.2 cm/sec.What is the rate of increase in the area of the olive oil when its circumference measures 20 $\pi$ cm?

Consider the two-parameter family of curves $y=\alpha x+\frac{b}{x}$ , with $a>0$ and $b>0$ .Is the graph concave up or down at the point x = -2?

A Library of Functions

Key Concept: the Derivative

Short-Cuts to Differentiation

Key Concept- the Definite Integral

Constructing Antiderivatives

Integration

Using the Definite Integral

Sequences and Series

Approximating Functions Using Series

Differential Equations

Functions of Several Variables

A Fundamental Tool- Vectors

Differentiating Functions of Several Variables

Optimization- Local and Global Extrema

Integrating Functions of Several Variables

Parameterization and Vector Fields

Line Integrals

Flux Integrals and Divergence

The Curl and Stokes Theorem

Parameters, Coordinates, Integrals

Filters

Exam 4: Using the Derivative

Starting at time t = 0, water is poured at a constant rate into an empty vase (pictured below).It takes ten seconds for the vase to be filled completely to the top.Let h = f(t)be the depth of the water in the vase at time t.For what value of h is f′(t)f^{\prime}(t)f′(t) the largest?

Given the function f(x)=x2e−2xf(x)=x^{2} e^{-2 x}f(x)=x2e−2x , then at x = 1 the graph of f has

Sketch a graph of a function whose f′(x)=0f^{\prime}(x)=0f′(x)=0 at x=1, f′(x)f^{\prime}(x)f′(x) < 0 when x< 1, f′(x)f^{\prime}(x)f′(x) < 0 when x> 1, Is it possible to have f′′(x)=0f^{\prime \prime}(x)=0f′′(x)=0 at any value from -2<x<2?

Motion of a particle is given by x=t2−3t,y=2t3−3tx=t^{2}-3 t, \quad y=2 t^{3}-3 tx=t2−3t,y=2t3−3t where t is time in minutes.Find the instantaneous speed of the particle at t = 3.Round to 2 decimal places.

Consider the function f(x)=ax(1−x)f(x)=a x(1-x)f(x)=ax(1−x) for a>0a>0a>0 .What is largest value of a such that f(x)≤3f(x) \leq 3f(x)≤3 on the region 0≤x≤10 \leq x \leq 10≤x≤1 ?

Graph the function f(x)=x2e−xf(x)=x^{2} e^{-x}f(x)=x2e−x for −1≤x≤3-1 \leq x \leq 3−1≤x≤3 .

A normal distribution in statistics is modeled by the function N(x)=12πe−x2/2N(x)=\frac{1}{\sqrt{2 \pi}} e^{-x^{2} / 2}N(x)=2π​1​e−x2/2 determine the limit of N(x)as x→x \rightarrowx→ - ∞\infty∞

In the function y=2 sin (x)+1.96, in the interval from 0 ≤x≤\leq x \leq≤x≤ π\piπ , at which value(s)of x does the function contain a global maximum?

Air is being blown into a spherical balloon at a rate of 70 cm3 per second.At what rate is the surface area of the balloon increasing when the radius is 10 cm? Round to 2 decimal places, and do not include units.

A water tank is constructed in the shape of a sphere seated atop a circular cylinder.If water is being pumped into the tank at a constant rate, let h(t)h(t)h(t) be the height of the water as a function of time.For the interval a < t < b, which of the following is true?

Let f(x)=e−x2bf(x)=e^{\frac{-x^{2}}{b}}f(x)=eb−x2​ , where b is a positive constant.Which of the following are inflection points of f?

A cupful of olive oil falls on the floor forming a circular puddle.Its radius is increasing at a constant rate of 0.2 cm/sec.What is the rate of increase in the area of the olive oil when its circumference measures 20 π\piπ cm?

Consider the two-parameter family of curves y=αx+bxy=\alpha x+\frac{b}{x}y=αx+xb​ , with a>0a>0a>0 and b>0b>0b>0 .Is the graph concave up or down at the point x = -2?

A Library of Functions

Key Concept: the Derivative

Short-Cuts to Differentiation

Key Concept- the Definite Integral

Constructing Antiderivatives

Integration

Using the Definite Integral

Sequences and Series

Approximating Functions Using Series

Differential Equations

Functions of Several Variables

A Fundamental Tool- Vectors

Differentiating Functions of Several Variables

Optimization- Local and Global Extrema

Integrating Functions of Several Variables

Parameterization and Vector Fields

Line Integrals

Flux Integrals and Divergence

The Curl and Stokes Theorem

Parameters, Coordinates, Integrals

Filters

Given the function $f(x)=x^{2} e^{-2 x}$ , then at x = 1 the graph of f has

Sketch a graph of a function whose $f^{\prime}(x)=0$ at x=1, $f^{\prime}(x)$ < 0 when x< 1, $f^{\prime}(x)$ < 0 when x> 1, Is it possible to have $f^{\prime \prime}(x)=0$ at any value from -2<x<2?

Motion of a particle is given by $x=t^{2}-3 t, \quad y=2 t^{3}-3 t$ where t is time in minutes.Find the instantaneous speed of the particle at t = 3.Round to 2 decimal places.

Consider the function $f(x)=a x(1-x)$ for $a>0$ .What is largest value of a such that $f(x) \leq 3$ on the region $0 \leq x \leq 1$ ?

Graph the function $f(x)=x^{2} e^{-x}$ for $-1 \leq x \leq 3$ .

A normal distribution in statistics is modeled by the function $N(x)=\frac{1}{\sqrt{2 \pi}} e^{-x^{2} / 2}$ determine the limit of N(x)as $x \rightarrow$ - $\infty$

In the function y=2 sin (x)+1.96, in the interval from 0 $\leq x \leq$ $\pi$ , at which value(s)of x does the function contain a global maximum?

Air is being blown into a spherical balloon at a rate of 70 cm³ per second.At what rate is the surface area of the balloon increasing when the radius is 10 cm? Round to 2 decimal places, and do not include units.

A water tank is constructed in the shape of a sphere seated atop a circular cylinder.If water is being pumped into the tank at a constant rate, let $h(t)$ be the height of the water as a function of time.For the interval a < t < b, which of the following is true?

Let $f(x)=e^{\frac{-x^{2}}{b}}$ , where b is a positive constant.Which of the following are inflection points of f?

A cupful of olive oil falls on the floor forming a circular puddle.Its radius is increasing at a constant rate of 0.2 cm/sec.What is the rate of increase in the area of the olive oil when its circumference measures 20 $\pi$ cm?

Consider the two-parameter family of curves $y=\alpha x+\frac{b}{x}$ , with $a>0$ and $b>0$ .Is the graph concave up or down at the point x = -2?