Exam 15: Random Variables
Exam 1: Data30 Questions
Exam 2: Displaying and Describing Categorical Data65 Questions
Exam 3: Displaying and Summarizing Quantitative Data93 Questions
Exam 4: Understanding and Comparing Distributions102 Questions
Exam 5: The Standard Deviation As a Ruler and the Normal Model131 Questions
Exam 6: Scatterplots, association, and Correlation74 Questions
Exam 7: Linear Regression57 Questions
Exam 8: Regression Wisdom32 Questions
Exam 9: Re-Expressing Data: Get It Straight51 Questions
Exam 10: Understanding Randomness26 Questions
Exam 11: Sample Surveys50 Questions
Exam 12: Experiments and Observational Surveys87 Questions
Exam 13: From Randomness to Probability64 Questions
Exam 14: Probability Rules90 Questions
Exam 15: Random Variables112 Questions
Exam 16: Probability Models114 Questions
Exam 17: Sampling Distribution Models45 Questions
Exam 18: Confidence Intervals for Proportions56 Questions
Exam 19: Testing Hypotheses About Proportions50 Questions
Exam 20: More About Tests69 Questions
Exam 21: Comparing Two Proportions52 Questions
Exam 22: Inferences About Means106 Questions
Exam 23: Comparing Means43 Questions
Exam 24: Paired Samples and Blocks33 Questions
Exam 25: Comparing Counts78 Questions
Exam 26: Inferences for Regression51 Questions
Exam 27: Analysis of Variance39 Questions
Exam 28: Multifactor Analysis of Variance22 Questions
Exam 29: Multiple Regression22 Questions
Exam 30: Multiple Regression Wisdom21 Questions
Exam 31: Rank-Based Nonparametric Tests29 Questions
Exam 32: The Bootstrap31 Questions
Select questions type
You have arranged to go camping for two days in March.You believe that the probability that it will rain on the first day is 0.5.If it rains on the first day,the probability that it also rains on the second day is 0.7.If it doesn't rain on the first day,the probability that it rains on the second day is 0.2.Let the random variable X be the number of rainy days during your camping trip.Find the expected value of X.
(Multiple Choice)
4.8/5  (38)
(38)
Miguel buys a large bottle and a small bottle of juice.The amount of juice that the manufacturer puts in the large bottle is a random variable with a mean of 1,018 ml and a standard deviation of 9 ml.The amount of juice that the manufacturer puts in the small bottle is a random variable with a mean of 506 ml and a standard deviation of 3 ml.Find the mean and standard deviation of the total amount of juice in the two bottles.
(Multiple Choice)
4.9/5  (32)
(32)
A company selling vegetable seeds in packets of 40 estimates that the mean number of seeds that will actually grow is 35.7 with a standard deviation of 1.9 seeds.If a customer buys 5 different seed packets,what are the expected value and standard deviation of the number of bad seeds? Assume that packets are independent of each other.
(Multiple Choice)
4.7/5  (36)
(36)
An insurance company estimates that it should make an annual profit of $150 on each homeowner's policy written,with a standard deviation of $6,700.If it writes 12,469 of these policies,what is the probability that the company will be profitable? Assume that policies are independent of each other and that the company's total profit follows a Normal model.
(Multiple Choice)
4.7/5  (33)
(33)
An insurance company estimates that it should make an annual profit of $150 on each homeowner's policy written,with a standard deviation of $6000.If it writes three of these policies,the mean annual profit for the company is found to be 3 × 150 = 450.The standard deviation of the company's annual profit is found to be 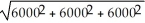 ≈ $10,392.What assumptions (if any)underlie the calculation of the mean? of the standard deviation?
≈ $10,392.What assumptions (if any)underlie the calculation of the mean? of the standard deviation?
(Multiple Choice)
4.9/5  (44)
(44)
A slot machine at a casino pays out an average of $0.90,with a standard deviation of $120.It costs a dollar to play.If gamblers play this machine 6,969,600 times in a month,what is the probability that the casino will come out ahead? Assume that the casino's total profit follows a Normal model.
(Multiple Choice)
4.7/5  (34)
(34)
The probabilities that a batch of 4 computers will contain 0,1,2,3,and 4 defective computers are 0.4746,0.3888,0.1195,0.0163,and 0.0008,respectively.Find the standard deviation of the number of defective computers.
(Multiple Choice)
4.8/5  (35)
(35)
Hugh buys $8,000 worth of stock in an electronics company which he hopes to sell afterward at a profit.The company is developing a new laptop computer and a new desktop computer.If it releases both computers before its competitor,the value of Hugh's stock will jump to $22,000.If it releases one of the computers before its competitor,the value of Hugh's stock will jump to $16,000.If it fails to release either computer before its competitor,Hugh's stock will be worth only $5,000.Hugh believes that there is a 70% chance that the company will release the laptop before its competitor and a 60% chance that the company will release the desktop before its competitor.Find Hugh's expected profit.Assume that the development of the laptop and the development of the desktop are independent events.
(Multiple Choice)
5.0/5  (29)
(29)
A company is interviewing applicants for managerial positions.They plan to hire two people.They have already rejected most candidates and are left with a group of 10 applicants of whom 4 are women.Unable to differentiate further between the applicants,they choose two people at random from this group of 10.Let the random variable X be the number of men that are chosen.Find the expected value of X.
(Multiple Choice)
4.7/5  (32)
(32)
A couple plans to have children until they get a boy,but they agree that they will not have more than four children even if all are girls.Find the expected number of children they will have.Assume that boys and girls are equally likely.Round your answer to three decimal places.
(Multiple Choice)
4.7/5  (34)
(34)
Sue buys a large packet of rice.The amount of rice that the manufacturer puts in the packet is a random variable with a mean of 1,016 g and a standard deviation of 8 g.The amount of rice that Sue uses in a week has a mean of 200 g and a standard deviation of 29 g.If the weight of the rice remaining in the packet after a week can be described by a normal model,what's the probability that the packet still contains more than 831.0 g after a week?
(Multiple Choice)
4.8/5  (36)
(36)
You pick a card from a deck.If you get a face card,you win $15.If you get an ace,you win $20 plus an extra $40 for the ace of hearts.For any other card you win nothing.Find the standard deviation of the amount you will win.
(Multiple Choice)
4.9/5  (32)
(32)
Jo is a hairstylist.The probability model below describes the number of clients that she may see in a day. 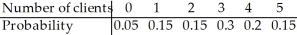 What is the standard deviation of the number of clients that Jo sees per day?
What is the standard deviation of the number of clients that Jo sees per day?
(Multiple Choice)
4.8/5  (28)
(28)
The accompanying table describes the probability distribution for the number of adults in a certain town (among 4 randomly selected adults)who have a degree from a post-secondary institute.Find the standard deviation for the probability distribution. 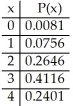
(Multiple Choice)
4.7/5  (41)
(41)
Given independent random variables with means and standard deviations as shown,find the mean and standard deviation of the variable 4Y - 5.Round to two decimal places if necessary. 
(Multiple Choice)
4.9/5  (29)
(29)
A carnival game offers a $80 cash prize for anyone who can break a balloon by throwing a dart at it.It costs $5 to play and you're willing to spend up to $20 trying to win.You estimate that you have an 10% chance of hitting the balloon on any throw.Create a probability model for the number of darts you will throw.Assume that throws are independent of each other.Round to four decimal places if necessary.
(Multiple Choice)
4.9/5  (32)
(32)
An insurance policy costs $400,and will pay policyholders $10,000 if they suffer a major injury (resulting in hospitalization),or $2,000 if they suffer a minor injury (resulting in lost time from work).The company estimates that each year 1 in every 3,000 policyholders may have a major injury,and 1 in 1,000 a minor injury.Create a probability model for the company's profit on this policy.
(Multiple Choice)
4.9/5  (39)
(39)
The amount of money that Maria earns in a week is a random variable with a mean of $980 and a standard deviation of $25.The amount of money that Elena earns in a week is a random variable with a mean of $800 and a standard deviation of $10.
You would like to use a Normal model to determine the probability that Maria's weekly income is at least $233.85 more than Elena's weekly income (the probability that the difference M - E is at least $233.85).
Which of the following assumptions are needed?
A: Maria's weekly earnings are independent of Elena's weekly earnings.
B: Maria's weekly earnings and Elena's weekly earnings follow a Normal model.
C: Maria's weekly earnings are greater than Elena's weekly earnings.
(Multiple Choice)
4.9/5  (37)
(37)
In a box of 8 batteries,5 are dead.You choose two batteries at random from the box.Let the random variable X be the number of good batteries you get.Find the standard deviation of of X.
(Multiple Choice)
4.8/5  (46)
(46)
A company is interviewing applicants for managerial positions.They plan to hire two people.They have already rejected most candidates and are left with a group of 9 applicants of whom 4 are women.Unable to differentiate further between the applicants,they choose two people at random from this group of 9.Let the random variable X be the number of men that are chosen.Find the probability model for X.
(Multiple Choice)
5.0/5  (32)
(32)
Showing 61 - 80 of 112
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)