Exam 9: Large-Sample Tests of Hypotheses
Exam 1: Describing Data With Graphs94 Questions
Exam 2: Describing Data With Numerical Measures186 Questions
Exam 3: Describing Bivariate Data35 Questions
Exam 4: Probability and Probability Distributions136 Questions
Exam 5: Several Useful Discrete Distributions129 Questions
Exam 6: The Normal Probability Distribution196 Questions
Exam 7: Sampling Distributions162 Questions
Exam 8: Large-Sample Estimation173 Questions
Exam 9: Large-Sample Tests of Hypotheses210 Questions
Exam 10: Inference From Small Samples261 Questions
Exam 11: The Analysis of Variance156 Questions
Exam 12: Linear Regression and Correlation165 Questions
Exam 13: Multiple Regression Analysis178 Questions
Exam 14: Analysis of Categorical Data136 Questions
Exam 15: Nonparametric Statistics198 Questions
Select questions type
An insurance company wants to test the hypothesis that the mean amount of life insurance held by professional men equals that held by professional women. Accordingly, two independent simple random samples are taken from appropriate professional listings of men and women. The sample of 200 men reveals a mean amount of $140,000 with a standard deviation $26,000. The sample of 400 women shows a mean amount of $128,000 with a standard deviation of $3,000.
Test the hypothesis using a 0.05 significance level.
Test statistic = ______________
p-value = ______________
Critical Value(s) = ______________
Conclusion: ______________
Interpretation: __________________________________________
Construct 95% confidence interval for the difference in mean amount of life insurance held by professional men and women.
______________
Explain how to use the 95% confidence interval to test the appropriate hypotheses at 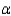 = 0.05.
__________________________________________
= 0.05.
__________________________________________
(Essay)
4.7/5  (43)
(43)
Let 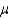 denote the true average delivery time of a letter from a specific carrier. For a large-sample z test of H0:
denote the true average delivery time of a letter from a specific carrier. For a large-sample z test of H0: 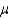 = 3 versus Ha:
= 3 versus Ha: 
 3, find the p-value associated with each of the given values of the test statistic, and state whether each p-value will lead to a rejection of the null hypothesis when performing a level 0.05 test.
3, find the p-value associated with each of the given values of the test statistic, and state whether each p-value will lead to a rejection of the null hypothesis when performing a level 0.05 test. 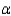 = 2.16
p = ______________
Conclusion: ______________
= 2.16
p = ______________
Conclusion: ______________ 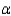 = 0.38
p = ______________
Conclusion: ______________
= 0.38
p = ______________
Conclusion: ______________ 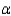 = -2.81
p = ______________
Conclusion: ______________
= -2.81
p = ______________
Conclusion: ______________ 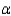 = 1.07
p = ______________
Conclusion: ______________
= 1.07
p = ______________
Conclusion: ______________ 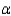 = -0.68
p = ______________
Conclusion: ______________
= -0.68
p = ______________
Conclusion: ______________
(Short Answer)
4.8/5  (39)
(39)
In testing 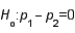 vs.
vs. 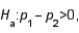 the test statistic value is found to be z = 1.28. The p-value of the test is approximately .1003.
the test statistic value is found to be z = 1.28. The p-value of the test is approximately .1003.
(True/False)
4.8/5  (32)
(32)
When the necessary conditions are met, an upper tailed test is being conducted for the difference between two population proportions. If the value of the test statistic is 2.90, then the p-value is 0.0038.
(True/False)
4.8/5  (33)
(33)
The width of a confidence interval estimate for a proportion will be:
(Multiple Choice)
4.7/5  (33)
(33)
The lower limit of the 90% confidence interval for the population proportion p, given that n = 400 and 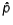 = 0.10 is 0.1247.
= 0.10 is 0.1247.
(True/False)
4.9/5  (30)
(30)
Which of the following statements about the null hypothesis is false?
(Multiple Choice)
4.8/5  (36)
(36)
A quality control officer tests bottles of shampoo to see if the filling machines are putting the proper amount in each bottle. They do not want to shut down production unless there is strong evidence indicating that the machines are not functioning properly. After testing a sample of bottles, the quality control officer decides to leave the filling machines operating. Actually, however, the filling machines are not operating properly. Which type of error, if any, did the quality control officer commit?
(Multiple Choice)
4.9/5  (40)
(40)
From a sample of 400 items, 14 are found to be defective. The point estimate of the population proportion defective will be:
(Multiple Choice)
4.9/5  (23)
(23)
Showing 201 - 210 of 210
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)