Exam 9: Large-Sample Tests of Hypotheses

A toaster manufacturer receives large shipments of thermal switches from a supplier. A sample from each shipment is selected and tested. The manufacturer is willing to send the shipment back if the proportion of defective switches is more than 5%. Otherwise, the shipment will be kept. State the appropriate null and alternative hypotheses to be tested by the manufacturer. H₀: ______________ H₁: ______________ Describe the Type I error. ________________________________________________________ Describe the Type II error for this problem. ________________________________________________________ From the manufacturer's point of view, which error would be the more serious? ______________ Justify your answer. ________________________________________________________ From the supplier's point of view, which error would be the more serious? ______________ Justify your answer. ________________________________________________________

Historically, the average time it takes Jessica to swim the 200 meter butterfly is 148.4 seconds. Jessica would like to know if her average time has changed. She records her time on 50 randomly selected occasions and computes the mean to be 147.8 seconds with a standard deviation of 2.3 seconds. Perform the appropriate test of hypothesis to determine whether Jessica's average time has changed. Use = 0.01. Test statistic = ______________ Critical Value(s) = ______________ Conclusion: ______________ Interpretation: __________________________________________ Compute the power of the test if Jessica's actual mean swimming time is 147.3 seconds. ______________ Interpret the results. ________________________________________________________

In clinical studies of an allergy drug, 81 of the 900 subjects experienced drowsiness. A competitor claims that 10% of the users of this drug experience drowsiness. Is there enough evidence at the 5% significance level to infer that the competitor is correct? Test statistic = ______________ p-value = ______________ Conclusion: ______________ Interpretation: __________________________________________ Construct a 95% confidence interval estimate of the population proportion of the users of this allergy drug who experience drowsiness. ______________ Explain how to use this confidence interval to test the hypotheses. __________________________________________

Which of the following would be an appropriate alternative hypothesis?

If the null hypothesis is actually false, then a hypothesis test may result in a Type II error.

The proportion of defective computers built by Byte Computer Corporation is 0.15. In an attempt to lower the defective rate, the owner ordered some changes made in the assembly process. After the changes were put into effect, a random sample of 42 computers were tested revealing a total of 4 defective computers. Perform the appropriate test of hypothesis to determine whether the proportion of defective computer has been lowered. Use = 0.01. Test statistic = ______________ Critical Value(s) = ______________ Conclusion: ______________ Interpretation: __________________________________________

The use of the standard normal distribution for constructing confidence interval estimate for the population proportion p requires:

In testing the difference between two population means using two independent samples, the population standard deviations are assumed to be known and the calculated test statistic equals 2.56. If the test is two-tail and 5% level of significance has been specified, the conclusion should be to:

An alternative hypothesis that holds for deviations from the null hypothesis in either direction is a two-sided hypothesis.

In a two-tailed test for the population mean, the null hypothesis will be rejected at 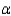level of significance if the value of the test statistic z is such that:

A Type I error is committed when we:

A set consisting of values that support the alternative hypothesis and lead to rejecting the null hypothesis is called the rejection region.

A hypothesis that specifies a single value for the unknown parameter is called a point estimate.

The power of a statistical test, denoted by 1 , is the probability of rejecting a false null hypothesis.

If a hypothesis test leads to incorrectly accepting the null hypothesis, a Type I error has been committed.

When we test for differences between the means of two independent populations, we can only use a two-tailed test.

A university investigation was conducted to determine whether women and men complete medical school in significantly different amounts of time, on the average. Two independent random samples were selected and the following summary information concerning times to completion of medical school computed: Perform the appropriate test of hypothesis to determine whether there is a significant difference in time to completion of medical school between women and men. Test using 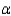= 0.05. Test statistic = ______________ Critical Value(s) = ______________ Conclusion: ______________ Interpretation: __________________________________________ Find the p-value associated with the test above. p-value = ______________

A two-tailed hypothesis test of the population mean is used when the alternative hypothesis takes the form .

To test the theory that the consumption of red meat in the United States has decreased over the last 10 years, a researcher decides to select hospital nutrition records for 400 subjects surveyed 10 years ago and to compare their average amount of beef consumed per year to amounts consumed by an equal number of subjects interviewed this year. The data are given in the table. 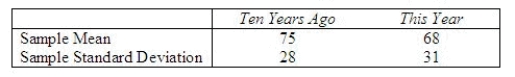Do the data present sufficient evidence to indicate that per-capita beef consumption has decreased in the last 10 years? Test at the 1% level of significance. Test statistic = ______________ Critical Value(s) = ______________ Conclusion: ______________ Interpretation: __________________________________________ Find a 99% lower confidence bound for the difference in the average per-capita beef consumption for the two groups. ______________ Does your confidence bound confirm your conclusions above? ______________ Explain. __________________________________________ What additional information does the confidence bound give you? __________________________________________

In a criminal trial, a Type I error is committed when: