Exam 9: Large-Sample Tests of Hypotheses

In estimating the difference between two population means, if a 90% confidence interval includes zero, then we can be 90% certain that the difference between the two population means is zero.

An Internet server claimed that its users averaged 15 hours per week. To determine whether this was an overstatement, a competitor conducted a survey of 150 customers and found that the average time spent online was 13 hours per week with a standard deviation of 6.5 hours. Do the data provide sufficient evidence to indicate that the average hours of use are less than that claimed by the first Internet server? Test at the 1% level of significance. Test statistic = ______________ p-value = ______________ Conclusion: ______________ Interpretation: __________________________________________

Which of the following is a required condition for using the normal approximation to the binomial in testing the difference between two population proportions?

When testing for differences between the means of two dependent populations, we can use either a one-tailed or two-tailed test.

Independent random samples of 35 and 50 observations are drawn from two quantitative populations, 1 and 2, respectively. The sample data summary is shown here: 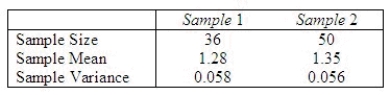Do the data present sufficient evidence to indicate that the mean for population 1 is smaller than the mean for population 2? Test statistic = ______________ Critical Value(s) = ______________ Conclusion: ______________ Interpretation: __________________________________________ Find the p-value for the test above. p-value = ______________

John Kerry believes that 54% of the voters are supporting him. His opponent, George W. Bush, believes this estimate is too high. State the appropriate null and alternative hypotheses to be tested by George W. Bush. H₀: ______________ H₁: ______________ Describe the Type I error for this problem. ________________________________________________________ Describe the Type II error for this problem. ________________________________________________________ Describe the practical consequences for George W. Bush if he makes a Type I error. ________________________________________________________ Describe the practical consequences for the George W. Bush if he makes a Type II error. ________________________________________________________

When testing , the observed value of the z-score was found to be -2.15. Then, the p-value for this test would be

In estimating the difference between two population means, the estimate for the standard deviation of the sampling distribution of 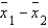is found by taking the square root of the sum of the two sample variances.

In testing the difference between two population means using two independent samples, the sampling distribution of the sample mean difference is normal if the sample sizes are both greater than 30.

In testing 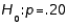vs. 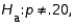random sample of size 200 produced a sample proportion 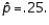Given these results, the p-value of the test is approximately:

If we reject the null hypothesis, we conclude that:

In testing 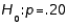vs. 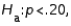a random sample of size 200 produced a sample proportion Given these results, the null hypothesis should not be rejected at 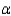= .05.

In a one-tailed test, the larger the significance level 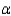, the larger the critical value will be.

In formulating the null and alternative hypothesis, which of the following would be an acceptable null hypothesis?

When testing , the observed value of the z-score was found to be -2.15. Then, the p-value for this test would be:

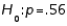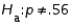In testing vs. at 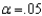, any p-value greater than .025 will lead to a rejection of the null hypothesis.

In hypothesis testing, the decision to "accept" the null hypothesis is the same as the decision to "fail to reject" the null hypothesis.

The p-value for a hypothesis test is set up by the decision maker to minimize the probability of committing a Type I error.

The campaign manager of John Kerry believes that more than 52% of the registered voters will vote in favor of Kerry. If you wish to test this claim, the appropriate null and alternative hypotheses are 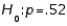vs. .

The p-value or observed significance level measures the strength of the evidence against the alternative hypothesis.